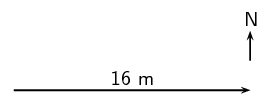
Classify the following quantities as scalars or vectors:
-
\(\text{12}\) \(\text{km}\)
-
\(\text{1}\) \(\text{m}\) south
-
\(\text{2}\) \(\text{m·s$^{-1}$}\), \(\text{45}\)\(\text{°}\)
-
\(\text{075}\)\(\text{°}\), \(\text{2}\) \(\text{cm}\)
-
\(\text{100}\) \(\text{km·h$^{-1}$}\), \(\text{0}\)\(\text{°}\)